Tag: concepts

Concepts and misconceptions are at the heart of maths education.  Our goal is to give out children an understanding of concepts that they trust and can use.  We may have to do this through knowledge and skills because (except in The Matrix) we cannot download whole concepts into people’s heads.  We can run into difficulties if we assume that certain concepts are in place based only on the assessment of a few key facts and skills.

AN EXAMPLE – MULTIPLYING BY 10

Let’s start with a simple one.  Lots of us either learn or realise that if we multiply whole numbers by 10, we can just add a zero to the end of the number to get the answer.  Most educators realise that this is a very limited trick but it allows children to develop ways to respond to questions before they can deal with the concept of place value.

The problem is that the technique suggests that you can just append numbers here and there.  It is a way to get a correct answer but not a mathematical operation that works in any other situation.

This type of problem is commonly seen in algebra when people talk about moving numbers or variables to the other side.  It’s not so bad to do this once you have a strong concept of algebra but before that, it tends to develop real misconceptions about how maths works.

ROBUST CONCEPTIONS OF MULTIPLICATION

If all you learn about multiplication is times tables and perhaps the column method, it won’t be too surprising if your concept of multiplication doesn’t go any further than repeated addition.  Repeated addition may be a form of multiplication but there is a lot more to it.  To have a robust internal conception of multiplication, you would want experiences of other ways that multiplication is used.  Natural Math have a great poster that shows 12 different models of multiplication. 

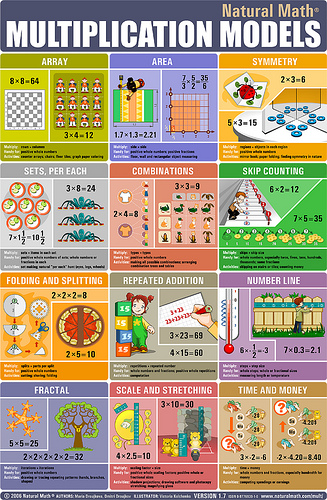
It is really important for developing understanding of more advanced topics, that your internal conception is able to accept all of these models.  In a way, you want your ideas to be open to more than the first technique that you learnt.  That way you form connections with other areas of maths and will be able to solve problems more creatively.

PRACTICES FOR BROADER CONCEPT OF MULTIPLICATION

Here are a few things that will really help you broaden the concept of multiplication for your children or students.

1) Try the Multiplication Explorers course by Natural Math

2) Use grids, tables and webs to model multiplication as well as lists

3) Practise with Prime Colours.  These numbers are coded according to the fundamental theorem of arithmetic and develop more robust thinking about multiplication by making the process visible and physical.